We have learnt about multiplication of two or more real numbers in the earlier lesson. You can very easily write the following.
4 × 4 × 4 = 64,11 × 11 × 11 × 11 = 14641
and 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 = 256
Think of the situation when 13 is to be multiplied 15 times. How difficult is it to write?
13 × 13 × 13 ×……………..15 times?
This difficulty can be overcome by the introduction of exponential notation. In this lesson, we shall explain the meaning of this notation, state and prove the laws of exponents and learn to apply these. We shall also learn to express real numbers as product of powers of prime numbers.
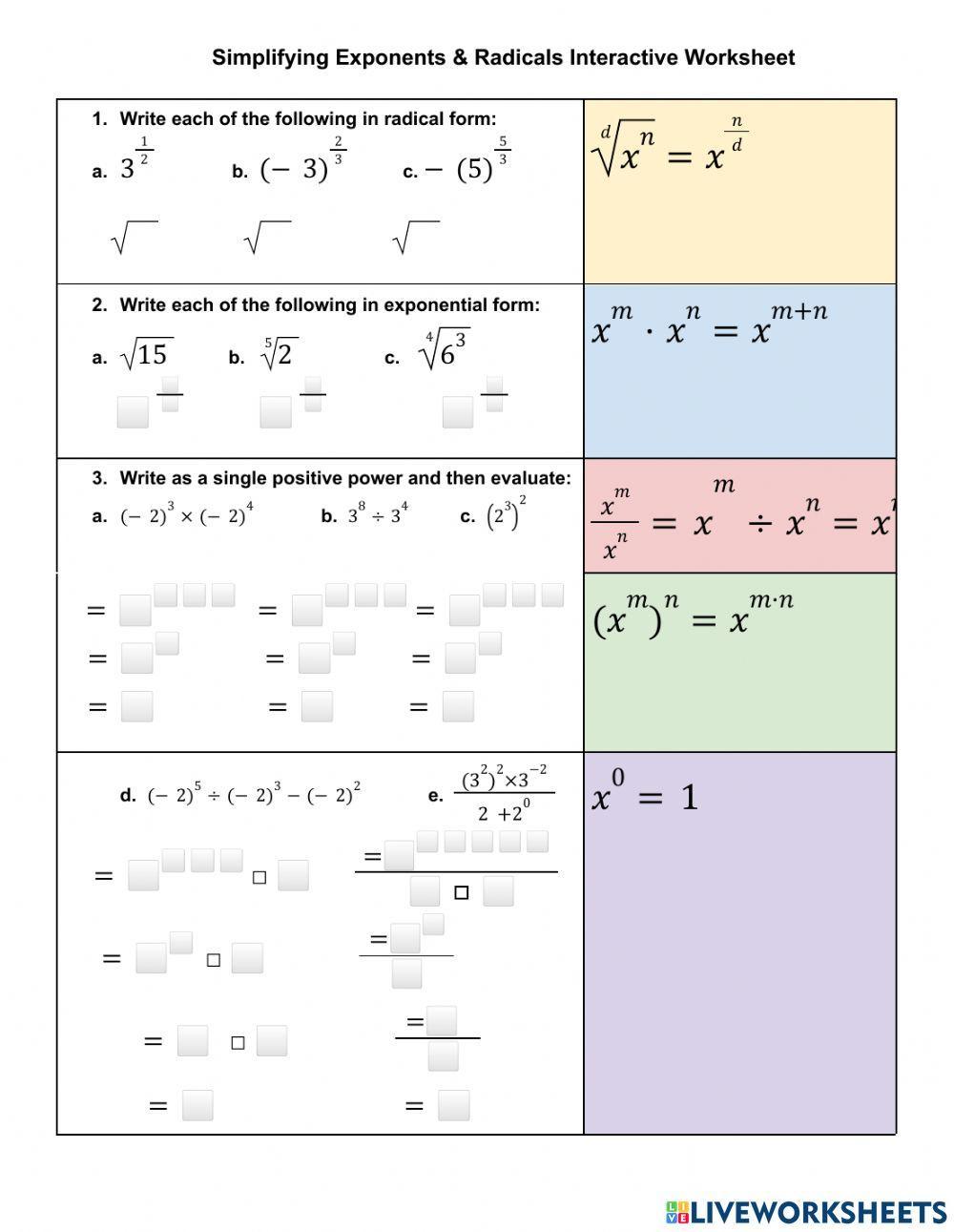
In the next part of this lesson, we shall give a meaning to the number a1/q as qth root of a. We shall introduce you to radicals, index, radicand etc. Again, we shall learn the laws of radicals and find the simplest form of a radical. We shall learn the meaning of the term “rationalising factor’ and rationalise the denominators of given radicals.
OBJECTIVES
After seen this video, you will be able to
• write a repeated multiplication in exponential notation and vice-versa;
• identify the base and exponent of a number written in exponential notation;
• express a natural number as a product of powers of prime numbers uniquely;
• state the laws of exponents;
• explain the meaning of a0, a–m and a p/q;
• simplify expressions involving exponents, using laws of exponents;
• write a repeated multiplication in exponential notation and vice-versa;
• identify the base and exponent of a number written in exponential notation;
• express a natural number as a product of powers of prime numbers uniquely;
• state the laws of exponents;
• explain the meaning of a0, a–m and a p/q;
• simplify expressions involving exponents, using laws of exponents;
EXPECTED BACKGROUND KNOWLEDGE
• Prime numbers
• Four fundamental operations on numbers
• Rational numbers
• Order relation in numbers.
EXPONENTIAL NOTATION


Example 2.1:Evaluate each of the following:

Example 2.3: Express each of the following in exponential notation and write the base and exponent in each case.





PRIME FACTORISATION
Recall that any composite number can be expressed as a product of prime numbers. Let us take the composite numbers 72, 760 and 7623.











NEGATIVE INTEGERS AS EXPONENTS


LAWS OF EXPONENTS FOR INTEGRAL EXPONENTS
After giving a meaning to negative integers as exponents of non-zero rational numbers, we can see that laws of exponents hold good for negative exponents also.
For example.


2.Express as a power of rational number with positive exponent:

MEANING OF ap/q



Solution:

CHECK YOUR PROGRESS 2.5


2.8 PURE AND MIXED SURD


Solution:
- index is 5 and radicand is 117.
- index is 2 and radicand is 162.
- index is 4 and radicand is 213.
- index is 4 and radicand is 214.
Example 2.18:Identify “pure” and “mixed” surds from the following:

2.10 LAWS OF RADICALS






2.13 SIMPLEST (LOWEST) FORM OF A SURD
A surd is said to be in its simplest form, if it has
a) smallest possible index of the sign
b) no fraction under radical sign
c) no factor of the form an , where a is a positive integer, under the radical sign of index n.

Solution:

CHECK YOUR PROGRESS 2.7

2.14 FOUR FUNDAMENTAL OPERATIONS ON SURDS
2.14.1 Addition and Subtraction of Surds


CHECK YOUR PROGRESS 2.8

2.14.2 Multiplication and Division in Surds
Two surds can be multiplied or divided if they are of the same order. We have read that the order of a surd can be changed by multiplying or dividing the index of the surd and index of the radicand by the same positive number. Before multiplying or dividing, we change them to the surds of the same order.
Let us take some examples:



2.15 COMPARISON OF SURDS
To compare two surds, we first change them to surds of the same order and then compare
their radicands along with their co-efficients. Let us take some examples:

CHECK YOUR PROGRESS 2.9

2.16 RATIONALISATION OF SURDS

In other words, the process of converting surds to rational numbers is called rationalisation
and two numbers which on multiplication give the rational number is called the rationalisation
factor of the other.





LET US SUM UP

• Operations on surds

